Institute
for Christian Teaching
Education
Department of Seventh-day Adventist
GOD AND CALCULUS
by
Norie Grace Rivera-Poblete
Mission College
Muak Lek Saraburi, Thailand
450-00 Institute for Christian Teaching
12501 Old Columbia Pike
Silver Spring, MD 20904 USA
Prepared for the
27th International Faith and Learning Seminar
held at
Mission, Muak Lek Saraburi, Thailand
December 3 – 15, 2000
Introduction
Calculus is one of the greatest achievements
of the human intellect. Sometimes
called the " mathematics of changes", it is the branch of mathematics
that deals with the precise way in which changes in one variable relate to
changes in another. In our daily
activities we encounter two types of variables: those that we can control directly and those that we cannot. Fortunately, those variables that we cannot
control directly often respond in some way to those we can. For example, the acceleration of a car
responds to the way in which we control the flow of gasoline to the engine; the
inflation rate of an economy responds to the way in which the national
government controls the money supply; and the level of antibiotics in a person's
bloodstream responds to the dosage and timing of a doctor's prescription. By understanding quantitatively how the
variables, which we cannot control directly, respond to those that we can, we
can hope to make predictions about the behavior of our environment and gain
some mastery over it. Calculus is one
of the fundamental mathematical tools used for this purpose.
Calculus was invented to answer questions
that could not be solved by using algebra or geometry. One branch of calculus, called
Differential calculus, begins with a question about the speed of moving
objects. For example, how fast does a
stone fall two seconds after it has been dropped from a cliff? The other branch of calculus, Integral
calculus, was invented to answer a very different kind of question: what is the area of a shape with curved
sides? Although these branches began by
solving different problems, their methods are the same, since they deal with
the rate of change.
Some anticipations of calculus can be seen in Euclid and other classical writers, but most of the ideas appeared first in the seventeenth century. Sir Isaac Newton (1642 – 1727) and Gottfried W. Leibniz (1646 – 1716) independently discovered the fundamental theorem of calculus. After its start in the seventeenth century, calculus went for over a century without a proper axiomatic foundation. Newton wrote that calculus could be rigorously founded on the idea of limits, but he never presented his ideas in detail. A limit, roughly speaking, is the value approached by a function near a given point. During the eighteenth century many mathematicians based their work on limits, but their definition of limit was not clear. In 1784, Joseph Louis Lagrange (1736-1813) at the Berlin Academy proposed a prize for a successful axiomatic foundation for calculus. He and others were interested in being as certain of the internal consistency of calculus as they were about algebra and geometry. No one was able to successfully respond to the challenge. It remained for Augustin Louis Cauchy (1789-1857) to show, sometime around 1820, that the limits can be defined rigorously by means of inequalities (Hughes-Hallett, 1998, 78).
Purpose of the study
Calculus is one of the subjects being taught
for higher mathematics in high schools and colleges. The purpose of this paper is to show how to use calculus in our
relationship with God. I will employ parallelism and contrast to teach the
values with the hope that through teaching calculus the teacher can bring
his/her students closer to God.
Application
¨
God is the
greatest mathematician. According to
Avery J. Thompson, "Any credence given to the study of mathematics must
recognize that God is the original mathematician. And though, through the ages, humankind has experimented to be
able to draw conclusion in the areas of mathematics, God's laws are error-free
and constant. His everlasting
watch-care in the 'natural' cyclic phenomena of this earth daily proves His
mathematical supremacy. Galileo is
remembered for having acknowledged that 'mathematics is the language that God
used to create the universe'".
¨
We are the
variables and God is the constant. God
doesn't change; He is the same God from the beginning. According to Malachi 3:6 (NIV) "I the
Lord do not change." As variables,
we depend on Him to give some predictability to life. Without some constancy, we would never be able to plan, or hope,
or know what to expect. God's laws,
both the moral law and the laws of nature, are as constant as He is. So we can expect that tomorrow the sun will
rise in the east, as it has done every day in the past.
¨
A given value
(constant) helps in solving a given function.
For example, it is estimated that x months from now, the population of a
certain community will be ![]() (function). At what rate will the population be changing
with respect to time 15 months (constant) from now? Solution: the rate of
change of the population with respect to time is the derivative of the
population function. That is,
(function). At what rate will the population be changing
with respect to time 15 months (constant) from now? Solution: the rate of
change of the population with respect to time is the derivative of the
population function. That is, ![]() . The rate of change
of the population 15 months from now will be
. The rate of change
of the population 15 months from now will be ![]() people per month.
God, who we said is constant, is a "present help in time of trouble"(Psalm
46:1). Indeed, without God in one's
life, we will never find satisfactory solutions to problems.
people per month.
God, who we said is constant, is a "present help in time of trouble"(Psalm
46:1). Indeed, without God in one's
life, we will never find satisfactory solutions to problems.
¨
In calculus, if
we violate the laws we will never find the right solution to the given problem
or function. When we violate the laws
of God our life become chaotic and we will never find peace or the right
solutions to our problems.
Limit and Limitless God
The
concept of limits is very important in calculus. Without limits calculus simply does not exist. Every single notion of calculus is a limit
in one sense or another. On the
contrary God has no limit. When we
apply the concept of limit, we examine what happens to the y-values of a function
f(x) as x gets closer and closer to
(but does not reach) some particular number, called a. If the y-values also get closer and closer
to a single number, L, then the number L is said to
be the limit of the function as x approaches a. Thus, we say that L is the limit of
f(x) as x approaches a. This is
written in mathematical shorthand as ![]()
where the symbol → stands for the word approaches.
If the y-values of the function do not get closer and closer to a single number
as x gets closer and closer to a, then the function has no limit
as x approaches a.
Figure 1 shows the graph of a function that has a limit L
as x approaches a particular a.
Illustration:
![]()
![]()
![]()
|
X |
1.0 |
1.5 |
1.9 |
1.95 |
1.99 |
1.995 |
1.999 |
2.0 |
2.001 |
2.005 |
2.01 |
2.05 |
2.1 |
2.5 |
3.0 |
|
f(x) |
1.00 |
1.75 |
2.71 |
2.85 |
2.97 |
2.985 |
2.997 |
|
3.003 |
3.015 |
3.03 |
3.15 |
3.31 |
4.75 |
7.0 |
![]()
![]()
Left side right
side
Table 1
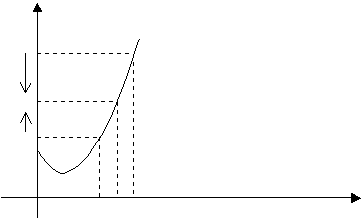 y
y
y = f(x)=x2-
x+1
f(x)
3
f(x)
x 2 x x
Figure 1
"Limit" reminds us of the experience of the Israelites, as they traveled through the wilderness. Most of the adult Israelites who came out from Egypt did not enter the Promised Land except for Caleb and Joshua. The children of Israel "approached" the Promised Land; generally speaking, all of them reached the border. But none of them would have made it were it not for God's limitless love and grace. Even though they disobeyed Him so many times, God still kept His covenant with the Israelites.
And thus, the limitless love of God is demonstrated in many other ways: in the parable of the lost sheep, the parable of the prodigal son, and even in the way God deals with His people today. Jeremiah 31: 3 (NIV) says: "I have loved you with an everlasting love." 2 Chronicles 16: 34 (NIV) says: "His love endures forever."
Let's define continuity. The idea of continuity rules out breaks, jumps, or holes by demanding that the behavior of the function near a point be consistent with its behavior at the point.
Definition: The function f is said to be continuous from a if and only if the following three conditions are satisfied:
i)
![]()
ii)
![]()
iii) ![]()
If one or more of these three conditions fail to hold at a, the function is said to be discontinuous at a.
Illustration: A wholesaler who sells a product by the kilogram (or fraction of
a kilogram) charges $2 per kilogram if 10 kg or less is ordered. If more than 10 kg is ordered, the
wholesaler charges $20 plus $1.40 for each kilogram in excess of 10 kg. Therefore, if x kilograms of the product is purchased at a total cost of ![]() dollars, then
dollars, then ![]() and
and ![]() that is,
that is,
![]()
Solution: i) ![]()
ii) ![]()
![]()
![]()
![]()
=![]() =
=![]()
iii) ![]()
Therefore C is continuous at 10.
We can now use this definition to represent our faith in God.
i)
If ![]() is our faith in God,
our faith in God exists
is our faith in God,
our faith in God exists
ii) ![]()
iii) ![]()
If any of the
above definitions does not exist, the function is said to be
discontinuous. One of the examples
showing that his faith in God was present was Moses when he led the Israelites
out in the desert. Moses and the people
were in the desert, but what was he going to do with them? They had to be fed and feed was what he did.
Moses needed to have around 1500 tons of food each day. To bring that much food each day, two
freight trains, each around a kilometer long would be needed. We all know they were out in the desert, so
they would need firewood to cook the food.
This would take 4000 tons of wood and a few more freight trains, each a
kilometer long, just for one day. And
they were forty years in the desert.
They would
have to have water. If they only had to
have enough to drink and wash a few dishes, it would take around 11,000,000
gallons each day, and a freight train with tank cars, around 3 kilometers long,
just to bring water.
Then another
thing: they had to cross the Red
Sea. Now, if they went on a narrow
path, double file, the line would be around 1200 km long and would take 35
days and nights to get
through. So, there had to be space in
the Red Sea, around 5 km wide so that they could walk 500 abreast to get over
in one night.
But then,
there was another problem. Each time
they camped at the end of the day, they needed a big space, a total of around
2000 square kilometers long.
Do you think
Moses sat down to figure all this out before he left Egypt? Moses believed in God, and that God would
take care of everything for him.
The following
persons also demonstrated faith in God: Noah, when God asked him to build the
ark, when they hadn't experienced flood or rain before that time; Abraham, when
God asked him to leave his family and go to another place and also when God
asked him to offer his only son Isaac.
To maintain a
living and growing relation with God we need to have continuous communication
with Him through prayer, meditation, and reading of His Word. We must have faith or trust in Him. According to Psalms 32: 10, "The Lord's
unfailing love surrounds the man who trusts in Him".
Great is our
Lord and mighty in power; his understanding has no limit. Ps. 147:5(NIV)
Derivative and Unchanging God
Derivative is
a mathematical tool that is used to study rate at which physical quantities
change. It is one of the two central
concepts of calculus, and it has a variety of applications, including curve
sketching, the optimization of functions, and the analysis of rates of change.
A typical problem to which calculus can be
applied is profit optimization. For
example: a manufacturer's monthly profit from the sale of radios was P(x)
= 400(15 – x)(x – 2) dollars when the radios were sold for x
dollars a piece. The graph of this
profit function, which is shown in Figure 2, suggests that there is an optimal
selling price x at which the manufacturer's profit will be
greatest. In geometric terms, the
optimal price is the x coordinate of the peak of the graph.
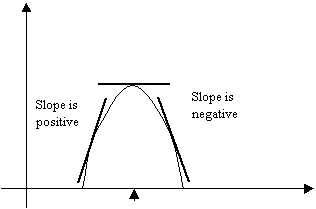 P(x)
P(x)
Slope
is zero
Optimal price
x
Figure 2:
The
profit function P(x) = 400(15 – x)(x
– 2)
In this example, the peak can be
characterized in terms of lines that are tangent to the graph. In particular, the peak is the only point on
the graph at which the tangent line is horizontal, that is, at which the slope
is zero. To the left of the peak, the slope of the tangent is positive. To the right of the peak the slope is
negative.
Calculus is also one of the
techniques used to find the rate of change function. The rate of change of a linear function with respect to its
independent variables is equal to the steepness or slope of its straight-line
graph. Besides, this steepness or rate
of change is constant.
We can solve optimization problems
and compute rates of change if we have a procedure for finding the slope of the
tangent to a curve at a given point.
We begin with a function f, and on its graph we choose a point (x,
f(x)). Refer to Figure 3. We choose a small number h
≠ 0 and on the graph mark the point (x + h, f(x + h)). Now we draw the secant line that passes
through these two points. The situation
is pictured in Figure 4, first with h > 0 and then with h
< 0.
As h tends to zero
from the right (Figure 4), the secant line tends to the limiting position
indicated by the dashed line, and tends to the same limiting position as h
tends to zero from the left. The line
at this limiting position is what we call " the tangent to the graph at
the point (x, f(x)).
Since
the approximating secant lines have slopes
(*) ![]() ,
,
we can expect the tangent line,
the limiting position of these secants, to have slope
(**) ![]()
While (*) measures the steepness
of the line that passes through the points (x. f(x)) and (x +h, f(x
+h)), (**) measures the steepness of the graph at (x, f(x)) and is
called the "slope of the graph."
We can now define
differentiation.
A function f is
said to be differentiable at x if and only if
![]() exists.
exists.
If this limit exists, it is
called the derivative of f at x, and is denoted by f'(x).
Notations: ![]()
![]()
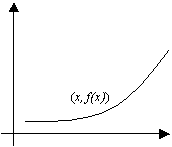 y
y
Figure 3 x
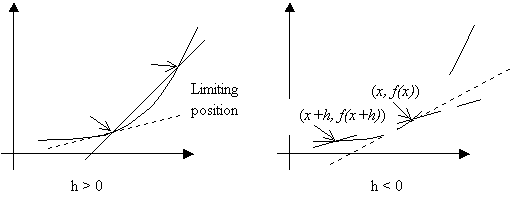 y y
y y
f f
Limiting position
Secant
(x+h, f(x+h))
(x,
f(x))
Secant
x x
Figure 4
Note: the rate of change of a function with respect to its independent variable is equal to the steepness of its graph, which is measured by the slope of its tangent line at the point in the question. Since the slope of the tangent line is given by the derivative of the function, it follows that the rate of change is equal to the derivative.
Illustrations:
1. It
is estimated that x months from now, the population of Mission College SDA
church will be ![]() At what rate will the
population be changing with respect to time 15 months from now?
At what rate will the
population be changing with respect to time 15 months from now?
Solution:
The rate of change of the population with respect to time is the derivative of the population function. That is
Rate of change = ![]()
The
rate of change of the population 15 months from now will be ![]() people per month
people per month
2.
|
Distance (S) |
Love (L) |
|
Velocity v= dS/dt |
God G = dL/dt = 0 |
Table 2
Since
the derivative of the constant is zero and we know that God's love is constant
and it does not change, so the rate of change of God's love is zero. 1 John 4:16(NIV) says: God is love. Hebrews 13: 8 says: "Jesus Christ (God) is the same
yesterday, today and forever."
Hence God's love is the same yesterday, today and forever. " There was enough, and more than
enough. In love there is no nice
calculation of less and more. God is like
that"(Barclay: p. 118)
Concavity and points of
Inflection:
The graph is concave up on an open interval where the slope increases and concave down on an open interval where the slope decreases. Points that join arcs of opposite concavity are called points of inflection. The graph in Figure 5 has three of them: (c1,f(c1)), (c2, f(c2)), (c3, f(c3)). In our daily lives we also have ups and downs and we have points of inflection, where we need to make decisions. According to Joshua 24: 15(NIV), "Choose for yourselves this day whom you will serve." We cannot serve two masters; one master brings us up and the other brings us down.
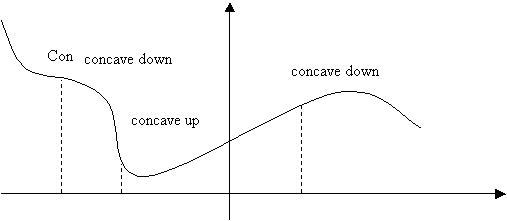 y
y
concave up

 x
x
Figure 5
Integral and
the Orderly God
In many problems, the derivative of a
function is known and the goal is to find the function itself. For example, a sociologist who knows the
rate at which the population is growing may wish to use this information to
predict future population levels; a physicist who knows the speed of a moving
body may wish to calculate the future position of the body; an economist who
knows the rate of inflation may wish to estimate future prices. God knows the rate of progress in His people
and He knows when His work will be completed, so He can reliably predict His
second coming, predict the close of probation, etc.
The process of obtaining a function from its
derivative is called antidifferentiation or integration.
Let's define Antiderivative:
A
function F(x) for which
![]()
for
every x in the domain of f is said to be an antiderivative ( or
indefinite integral) of f.
The Antiderivatives of a Function:
If F and G are
antiderivatives of f, then there is a constant C such that
![]()
Integral Notation: It is customary to write
![]()
to express the fact that every
antiderivative (integral) of f(x) is of the form F(x) + C. For example, we can express the fact that
every antiderivative of ![]() is of the form
is of the form ![]() by writing
by writing
![]()
The
symbol ![]() is called an integral sign and indicates that you are to find
the most general form of antiderivative or integral of the function following
it.
is called an integral sign and indicates that you are to find
the most general form of antiderivative or integral of the function following
it.
To apply integration we need to
follow specific rules, for example,
1.
The power rule
of Integrals; ![]() , for n
, for n![]() -1.
-1.
2. The Integral of ![]() ;
; ![]()
3. The Integral of ![]() ;
;![]()
4. The Constant Multiple Rule for Integrals;
![]()
5.
The Sum Rule
for Integrals; ![]()
There are more than 120 rules or properties
that can be used in Integration. There
is a specific rule that we apply for each kind of function. When God created this world, everything He
made had a specific role to play. For
example, the sun would give us light; air was for breathing; water was for
drinking and cleansing; trees, animals, and human beings were to live symbiotically;
gravity would keep everything from bouncing.
And He put everything in order.
God is a God of order. 1
Corinthians 14:33 (NIV) says, " God is not a God of disorder but of peace."
Suppose that the known the rate ![]() at which a certain
quantity F is changing and we wish to find the amount by which
the quantity F will change between x = a and x
= b. We would first find F
by antidifferentiation and then
at which a certain
quantity F is changing and we wish to find the amount by which
the quantity F will change between x = a and x
= b. We would first find F
by antidifferentiation and then
compute the difference. The
numerical result of such a computation is called definite integral of
the function f and is denoted by the symbol
![]() .
.
Applications
of Integrals:
1. Calculate the population of the town x months from now.
2. Total cost of producing x units.
3. Total consumption over the next t years.
4. Area
5. Volume up to three dimension
Illustration: Area in Polar Coordinate
![]()
The hardest part in applying
the Area formula is determining the limits (boundary) of integration. This can be done as follows:
1.
Sketch the
region R whose area is to be determined.
Genesis 2:7 (NIV) says: " God formed man of dust from the
ground."
2.
Draw an
arbitrary "radial line" from the pole to the boundary curve
![]()
Genesis
1:27 (NIV) says: " God created man in His own image"
3.
Ask, "Over
what interval of values must ![]() vary in order for the
radial line to sweep out the region R?"
vary in order for the
radial line to sweep out the region R?"
4.
Our answer in
Step 3 will determine the lower and upper limits of integration.
Psalms 8:5 (NIV) says: " Yet you have made him a little lower
than God."
Example:
Find the area
of the region in the first quadrant within the cardioid
r = 1 – cos![]()
Solution:
The region and a typical radial line are
shown in Figure 5. For the radial line
to sweep out the region, ![]() must vary from 0 to
must vary from 0 to ![]() .
.
![]()
![]()
![]()
![]()
To find the area of the region we
need to find the point where the radial line (point of reference). In order for us to find direction in our
life we need to have a point of reference, which is God.
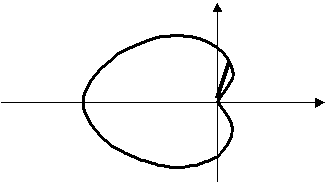 y
y
x
The shaded region is swept out by the
radial line as ![]() varies from 0 to
varies from 0 to ![]() .
.
Figure 5
Conclusion
From the above examples, we have shown some ways by which a mathematics teacher can use concepts in calculus to help students understand more about God. Calculus, or any subject, should not be taught in isolation but should be related to life and faith. True education should prepare a student not only for this life but also for the life to come. "True education means more than a preparation for the life that now is. It has to do with the whole being, and with the whole period of existence possible to the man. It is the harmonious development of the physical, the mental, and the spiritual powers. It prepares the student for the joy of the service in this world and for the higher joy of wider service in the world to come." (White, Counsels on Education) It should introduce the student to God and get him ready to enjoy His presence for eternity.
" Mathematics is a revelation of the
thought life of God. It shows Him to be
a God of system, order, and accuracy.
He can be depended upon. His
logic is certain. By thinking in
mathematical terms, therefore, we are actually thinking God's thoughts after
Him." (SDA, p.6)
References
- Anton,
Howard. Calculus A New Horizon. 6th ed. John Wiley, 1999.
- Barclay,
William. The Daily Study Bible
Series: the Gospel of Luke.
Westminster John Knox Press Louisville, Kentucky.
- Hoffmann,
Laurence and Bradley, Gerald. Brief
Calculus with applications. 5th
ed. McGraw Hill, 1993.
- Hughes-Hallett,
Gleason, McCallum, et al. Calculus,
Single and
Multiplevariable, 2nd ed.
John Willey, 1998
5. Holy Bible (NIV)
6.
Salas, Hille, and Etegen. Calculus
One and Several Variable. 8th
ed. John
Wiley, 1999.
7. SDA Secondary Curriculum (Mathematics).
- Thompson,
Avery J. "God in Nature:
Revelation of the Divine Mathematician." Christ in the Classroom.
Vol. 17.
- White, Ellen
G. Counsels on Education.
- White, Ellen
G. Education.